
|
Emil has a choice. He can buy a pair of sled runners for $37 at the local store, or he can buy a whole 1/4"x4'x8' sheet of UHMW 1 plastic in Anchorage for $220, cut it up, and bring it home. |
Subraction |
|

|
A pair of 2" runners is a total of 4" wide. He divided 4" into 48" and knew that he could get twelve pairs of runners. (He didn't count the saw kerf. He was just estimating at this point.) He divided 12 into $220 and got $18.33 for each pair. Then he got excited. How much would he save on each pair of runners if he cuts his own? |
Each space represents a pair of |
|
|
Disregard freight cost at this point. If he were to keep one pair of runners, and sell 11, letting the others pay for his runners, how much would he sell each pair at home in the village? How much profit would he make on the 11 pairs if he sold them for the store price of $37? (Subtract his cost from 11x$37) |
|||
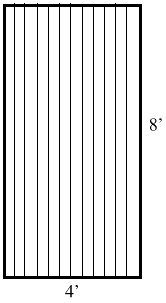
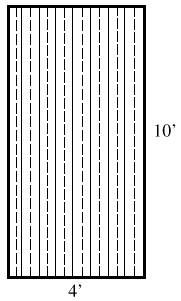
 If
he cut the 48" sheet into 3" runners, how much would he have
to sell the rest for in order to break even? (With his
runners free.)
If
he cut the 48" sheet into 3" runners, how much would he have
to sell the rest for in order to break even? (With his
runners free.)